Page 79 - LAPLACE TRANSFORM

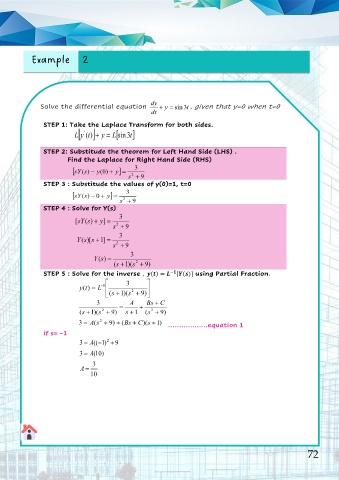
Example 2

dy
Solve the differential equation + y = sin t 3 , given that y=0 when t=0
dt
STEP 1: Take the Laplace Transform for both sides.

'
L [ ] yty )( + = [ L sin ] t 3

STEP 2: Substitude the theorem for Left Hand Side (LHS) .

Find the Laplace for Right Hand Side (RHS)
3
[sY (s ) − ) 0 ( y + y ] =
s 2 + 9

STEP 3 : Substitude the values of y(0)=1, t=0
3
[sY (s ) − 0 + y ] =
s 2 + 9
STEP 4 : Solve for Y(s)
3
[sY (s ) + y ] =
s 2 + 9
3
Y (s )[ + ] 1 =
s
s 2 + 9
3
Y (s ) = 2
s
( + 1 )(s + ) 9
STEP 5 : Solve for the inverse , ( ) = − [ ( )] using Partial Fraction.
 3 
y ) (t = L −1  2 
 (s + )(1 s + )9 
3 = A + Bs + C
( +s 1 )(s 2 + ) 9 s + 1 (s 2 + ) 9

3 = A (s 2 + ) 9 + (Bs + C )( +s ) 1 ………………equation 1
if s= -1
2
3 = A ((− ) 1 + 9
3 = ( A 10 )

3
=
A
10

72