Page 67 - LAPLACE TRANSFORM

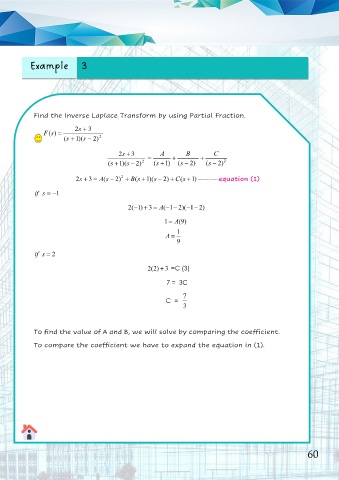
Example 3

Find the Inverse Laplace Transform by using Partial Fraction.

s
2 + 3
F (s ) =
s
s
( + 1 )( − ) 2 2
2 + 3 A B C
s
= + +
s
( + 1 )( − ) 2 2 ( + ) 1 ( − ) 2 ( − ) 2 2
s
s
s
s

2
2 +s 3 = A ( −s ) 2 + B ( +s 1 )( −s ) 2 + C ( +s ) 1 ----------equation (1)
if s = − 1
1−
(2 − ) 1 + 3 = A (− 2 )(− 1− ) 2
1= ) 9 ( A
1
A =
9

if s = 2

2(2 ) + 3 =C (3)

7 = 3C
7

C = 3

To find the value of A and B, we will solve by comparing the coefficient.

To compare the coefficient we have to expand the equation in (1).

60